Standard deviation

Table of Contents

Standard deviation
What is standard deviation?
Understanding standard deviation
Standard deviation formula
Calculating standard deviation
Using standard deviation
Frequently Asked Questions

Standard deviation

Investors use a statistical measurement to determine the market level, specific securities, or investment product volatility. This known as the standard deviation. It is among the most popularly used risk indicators, closely watched by professional and retail investors. It illustrates the size of differences between different values in a dataset. Investors would better comprehend the required rate of prospective returns by defining the level of risk.

What is standard deviation?

The standard deviation is a statistic that measures a dataset’s dispersion from its mean and is calculated as the square root of variance. Calculating each data point’s deviation from the normal may determine the standard deviation as the square root of variance.

The standard deviation is a statistical indicator of market volatility that quantifies how much prices fluctuate from the average price. If prices fluctuate within a narrow trading range, the standard deviation will show a low value, indicating low volatility.

Understanding standard deviation

The standard deviation is essential for figuring out how volatile an asset is. It displays how widely the asset’s price has changed over time. The higher the number, the more volatility you might expect. Investors can use the standard deviation as a starting point to assess the risks associated with a specific investment.

Investors can use the standard deviation measurement to guide how much trouble an item might bring to their portfolio, even if it only provides insight into historical performance. A fund with a higher standard deviation has more volatility. Returns on investments with a high standard deviation may vary either on the higher or lower side of the average and be characterised as inconsistent.

Future mutual fund monthly returns are predicted to fluctuate within one standard deviation of the average return 68% of the time and within two standard deviations 95% of the time.

Standard deviation formula

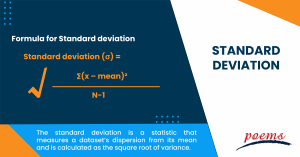
Standard deviation

Examining the variance between each data point and the average allows us to determine the standard deviation as the square root of variance.

Standard deviation (σ) = √[∑(x – mean)²/N-1]

Where,

x is the return observed in one period
mean is the arithmetic mean of the returns within the dataset
N is the dataset’s total number of observations.

Calculating standard deviation

Let’s calculate the standard deviation if, over five years, the returns for MNX retail services were 13.23%, 45.7%, 8.7%, 78.65%, and 56.8%.

The mean of returns is first solved by adding all values and dividing the result by the total number of values.

Mean of returns = 13.23 + 45.7 + 8.7 + 78.65 + 56.8 / 5 = 40.6

The variance is then calculated by adding all the values, combining the results, square rooting them, and dividing by the number of values minus one.

Variance = (13.23 – 40.6)2 + (45.7 – 40.6)2 + (8.7 – 40.6)2 + (78.65 – 40.6)2 – (56.8 – 40.6)2 / 5 -1 = 749.12 + 26.01 + 1,017.6 + 1,447.8 + 262.44 / 4 = 875.74.

Finding the square root of the variance yields the standard deviation, which produces a standard deviation of 29.6%.

Using standard deviation

The standard deviation is particularly useful in trading and investment methods since it is used to gauge the market’s volatility and securities and forecast performance trends. Given that their portfolio managers take risky bets to produce returns that are higher than average, one might anticipate aggressive growth funds to have a large standard deviation from relevant stock indices.

The standard deviation is among the most crucial basic risk gauges used by analysts, portfolio managers, and advisors. Investment companies disclose the standard deviation of mutual funds and other products.

Frequently Asked Questions

What is difference between standard deviation and variance?

The standard deviation is the range of a set of numbers from the mean. The variance calculates how far each point deviates from the norm on average. The variance, as opposed to the standard deviation, is the average of all the data points in a group.

While the variance is more valuable theoretically, the standard deviation is more beneficial to convey the variation in the data. For instance, the variance of the sum of the uncorrelated distributions (random variables) equals the total of the variances of the distributions.

What are the strengths of standard deviation?

The strengths of standard deviation are:

Standard deviation is a measure with a strict definition and a constant value.

A series of elements are used to calculate the standard deviation. It is the most accurate way to estimate dispersion.
Compared to other measurements, the standard deviation is least impacted by sample fluctuations, mean deviation, and quartile deviation.
Algebraic processes and procedures can make use of standard deviation. It is used in statistical analysis as well.

What are the limitations of standard deviation?

The limitations of standard deviation are:

Calculating the standard deviation is challenging compared to other methods of measuring dispersion.
When computing standard deviation, extreme values are given more weight than those close to the mean.
Open interval calculations are not possible for it.
Variations among such data sets are not compared if two or more data sets are provided in different units.

What is an example of standard deviation?

For example, consider Apple’s stock for a five-year term. Apple’s stores historically returned 12.49% in 2016, 48.45% in 2017, 5.39% in 2018, 88.98% in 2019, and 60.91% in 2020. Hence, 41.09% was the average return over the five years.

The annual return values were then, successively, -28.6%, 7.36%, -46.48%, 47.89%, and 19.82%. The results of square rooting all those values are 8.2%, 0.54%, 21.6%, 22.93%, and 3.93%. These values add up to 0.572.

To find the variance, multiply that number by 4 (N – 1), which equals (0.572/4) = 0.143. The standard deviation, which equals 0.3781, or 37.81%, is calculated by taking the square root of the variance.

How to find the standard deviation quickly?

To quickly determine the standard deviation, locate the mean. Calculate the standard deviation for each score. Any difference from the norm is squared. Determine the squares’ sum and find the difference. Determine the variance’s square root.